The Fundamental Theorem of Integral Calculus
Now we use the intuitive microcsope approximation (1.1.1) to prove:
Theorem: The Fundamental Theorem of Integral Calculus: Part 1
Suppose we want to find ![]() . If we can find another function
. If we can find another function ![]() so that the differential gives
so that the differential gives ![]() for every
for every ![]()
![]() , then
, then
![]()
The definition of the integral we use is the real number approximated by a sum of small slices,
![∫_a^bf[x] x≈Underoverscript[∑, Underscript[x = a, step δx], arg3] f[x] · δx](../HTMLFiles/Lect1_76.gif) ,when
,when ![]()
![[Graphics:../HTMLFiles/Lect1_78.gif]](../HTMLFiles/Lect1_78.gif)
Telescoping sums & derivatives
We know that if ![]() has derivative
has derivative ![]() , the differential approximation above says,
, the differential approximation above says,
![]()
so we can sum both sides
![]()
The telescoping sum satisfies,
![Underoverscript[∑, Underscript[x = a, step δx], arg3] F[x + δx] - F[x] = F[b '] - F[a]](../HTMLFiles/Lect1_83.gif)
so we obtain the approximation,
![]()
This gives,
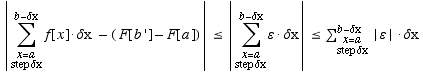
![≤ Max[| ε |] · Underoverscript[∑, Underscript[x = a, step δx], arg3] δx = Max[| ε |] · (b ' - a) ≈0](../HTMLFiles/Lect1_86.gif)
or ![∫_a^bf[x] x≈Underoverscript[∑, Underscript[x = a, step δx], arg3] f[x] · δx≈F[b '] - F[a]](../HTMLFiles/Lect1_87.gif) . Since
. Since ![]() is continuous,
is continuous, ![]() , so
, so ![]() .
.
We need to know that all the epsilons above are small when the step size is small, ![]() , when
, when ![]() for all
for all ![]() . This is a uniform condition that has a simple appearance in Robinson's theory. There is something to explain here because the theorem stated above is false if we take the usual pointwise notion of derivative and the Reimann integral. (There are pointwise differentiable functions whose derivative is not Riemann integrable.)
. This is a uniform condition that has a simple appearance in Robinson's theory. There is something to explain here because the theorem stated above is false if we take the usual pointwise notion of derivative and the Reimann integral. (There are pointwise differentiable functions whose derivative is not Riemann integrable.)
The condition needed to make this proof complete is natural geometrically and plays a role in the intuitive proof of the inverse function theorem in the next section.
Created by Mathematica (September 22, 2004)